alles zum Thema Quadratische Funktion.
Laden im
Google Play
Deutschland zwischen demokratie und diktatur
Europa und globalisierung
Demokratie und freiheit
Bipolare welt und deutschland nach 1953
Imperialismus und erster weltkrieg
Das 20. jahrhundert
Frühe neuzeit
Herausbildung moderner strukturen in gesellschaft und staat
Das geteilte deutschland und die wiedervereinigung
Europa und die welt
Friedensschlüsse und ordnungen des friedens in der moderne
Der mensch und seine geschichte
Die moderne industriegesellschaft zwischen fortschritt und krise
Akteure internationaler politik in politischer perspektive
Großreiche
Alle Themen

221
7
Anastasija
23.5.2021
Mathe
Quadratische Funktion
alles zum Thema Quadratische Funktion.
23.5.2021
4729

75
2276
9
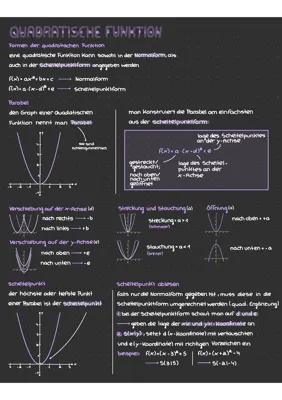
Quadratische Funktionsgleichungen
Normalform, Scheitelpunktform, umwandeln

114
3345
9/10
Lineare-, Quadratische-, Potenz- und Ganzrationale Funktionen
Steigung berechnen, Gleichung aufstellen, Funktionswert bestimmen, Nullstellen berechnen, Schnittpunkte bestimmenPunktsymmetrisch zum Ursprung, Achsensymmetrisch zur Y-Achse, Definitions- & Wertebereich, Scheitelpunkt- & Normalform, Globalverhalten
24
812
9/10
Quadratische Funktionen
Lernzettel über die Themen : Parabeln, Scheitelpunktform, Normalform und Nullstellen (pq-formel) aus der 9. Klasse auf einem G8 Gymnasium

96
2708
11/12
Kurvendiskussion Klausur
Eine Klausur der E2 mit dem Thema Kurvendiskussion - 15 NP (jedoch 2 kleine Fehler, welche aber erkennbar verbessert wurden)

41
1537
11/9
Funktionen
- Definition von Funktionen - Lineare Funktionen - Quadratische Funktionen - Potenzfunktionen

180
6819
11/12
ganzrationale funktionen und änderungsraten
selbstverfasster lernzettel zu - symmetrie von funktionen - verschieben, strecken, stauchen - durchschnittliche änderungsrate - substitution - linearfaktoren


Durchschnittliche App-Bewertung
Schüler:innen lieben Knowunity
In Bildungs-App-Charts in 17 Ländern
Schüler:innen haben Lernzettel hochgeladen
iOS User
Philipp, iOS User
Lena, iOS Userin
Anastasija
@anastasija.271
alles zum Thema Quadratische Funktion.

Zugriff auf alle Dokumente
Verbessere deine Noten
Werde Teil der Community
Mit der Anmeldung akzeptierst du die Nutzungsbedingungen und die Datenschutzrichtlinie
Unser KI-Begleiter ist speziell auf die Bedürfnisse von Schülern zugeschnitten. Basierend auf den Millionen von Inhalten, die wir auf der Plattform haben, können wir den Schülern wirklich sinnvolle und relevante Antworten geben. Aber es geht nicht nur um Antworten, sondern der Begleiter führt die Schüler auch durch ihre täglichen Lernherausforderungen, mit personalisierten Lernplänen, Quizfragen oder Inhalten im Chat und einer 100% Personalisierung basierend auf den Fähigkeiten und Entwicklungen der Schüler.
Du kannst dir die App im Google Play Store und im Apple App Store herunterladen.
Ja, du hast kostenlosen Zugriff auf Inhalte in der App und auf unseren KI-Begleiter. Zum Freischalten bestimmter Features in der App kannst du Knowunity Pro erwerben.
App Store
Google Play
Die App ist sehr leicht und gut gestaltet. Habe bis jetzt alles gefunden, nachdem ich gesucht habe und aus den Präsentationen echt viel lernen können! Die App werde ich auf jeden Fall für eine Klassenarbeit verwenden! Und als eigene Inspiration hilft sie natürlich auch sehr.
Stefan S
iOS user
Diese App ist wirklich echt super. Es gibt so viele Lernzettel und Hilfen, […]. Mein Problemfach ist zum Beispiel Französisch und die App hat mega viel Auswahl für Hilfe. Dank dieser App habe ich mich in Französisch verbessert. Ich würde diese jedem weiterempfehlen.
Samantha Klich
Android user
Wow ich bin wirklich komplett baff. Habe die App nur mal so ausprobiert, weil ich es schon oft in der Werbung gesehen habe und war absolut geschockt. Diese App ist DIE HILFE, die man sich für die Schule wünscht und vor allem werden so viele Sachen angeboten, wie z.B. Ausarbeitungen und Merkblätter, welche mir persönlich SEHR weitergeholfen haben.
Anna
iOS user
Ich finde Knowunity so grandios. Ich lerne wirklich für alles damit. Es gibt so viele verschiedene Lernzettel, die sehr gut erklärt sind!
Jana V
iOS user
Ich liebe diese App sie hilft mir vor jeder Arbeit kann Aufgaben kontrollieren sowie lösen und ist wirklich vielfältig verwendbar. Man kann mit diesem Fuchs auch normal reden so wie Probleme im echten Leben besprechen und er hilft einem. Wirklich sehr gut diese App kann ich nur weiter empfehlen, gerade für Menschen die etwas länger brauchen etwas zu verstehen!
Lena M
Android user
Ich finde Knowunity ist eine super App. Für die Schule ist sie ideal , wegen den Lernzetteln, Quizen und dem AI. Das gute an AI ist , dass er nicht direkt nur die Lösung ausspuckt sondern einen Weg zeigt wie man darauf kommt. Manchmal gibt er einem auch nur einen Tipp damit man selbst darauf kommt . Mir hilft Knowunity persönlich sehr viel und ich kann sie nur weiterempfehlen ☺️
Timo S
iOS user
Die App ist einfach super! Ich muss nur in die Suchleiste mein Thema eintragen und ich checke es sehr schnell. Ich muss nicht mehr 10 YouTube Videos gucken, um etwas zu verstehen und somit spare ich mir meine Zeit. Einfach zu empfehlen!!
Sudenaz Ocak
Android user
Diese App hat mich echt verbessert! In der Schule war ich richtig schlecht in Mathe und dank der App kann ich besser Mathe! Ich bin so dankbar, dass ihr die App gemacht habt.
Greenlight Bonnie
Android user
Ich benutze Knowunity schon sehr lange und meine Noten haben sich verbessert die App hilft mir bei Mathe,Englisch u.s.w. Ich bekomme Hilfe wenn ich sie brauche und bekomme sogar Glückwünsche für meine Arbeit Deswegen von mir 5 Sterne🫶🏼
Julia S
Android user
Also die App hat mir echt in super vielen Fächern geholfen! Ich hatte in der Mathe Arbeit davor eine 3+ und habe nur durch den School GPT und die Lernzettek auf der App eine 1-3 in Mathe geschafft…Ich bin Mega glücklich darüber also ja wircklich eine super App zum lernen und es spart sehr viel Heit dass man mehr Freizeit hat!
Marcus B
iOS user
Mit dieser App hab ich bessere Noten bekommen. Bessere Lernzettel gekriegt. Ich habe die App benutzt, als ich die Fächer nicht ganz verstanden habe,diese App ist ein würcklich GameChanger für die Schule, Hausaufgaben
Sarah L
Android user
Hatte noch nie so viel Spaß beim Lernen und der School Bot macht super Aufschriebe die man Herunterladen kann total Übersichtlich und Lehreich. Bin begeistert.
Hans T
iOS user
Die App ist sehr leicht und gut gestaltet. Habe bis jetzt alles gefunden, nachdem ich gesucht habe und aus den Präsentationen echt viel lernen können! Die App werde ich auf jeden Fall für eine Klassenarbeit verwenden! Und als eigene Inspiration hilft sie natürlich auch sehr.
Stefan S
iOS user
Diese App ist wirklich echt super. Es gibt so viele Lernzettel und Hilfen, […]. Mein Problemfach ist zum Beispiel Französisch und die App hat mega viel Auswahl für Hilfe. Dank dieser App habe ich mich in Französisch verbessert. Ich würde diese jedem weiterempfehlen.
Samantha Klich
Android user
Wow ich bin wirklich komplett baff. Habe die App nur mal so ausprobiert, weil ich es schon oft in der Werbung gesehen habe und war absolut geschockt. Diese App ist DIE HILFE, die man sich für die Schule wünscht und vor allem werden so viele Sachen angeboten, wie z.B. Ausarbeitungen und Merkblätter, welche mir persönlich SEHR weitergeholfen haben.
Anna
iOS user
Ich finde Knowunity so grandios. Ich lerne wirklich für alles damit. Es gibt so viele verschiedene Lernzettel, die sehr gut erklärt sind!
Jana V
iOS user
Ich liebe diese App sie hilft mir vor jeder Arbeit kann Aufgaben kontrollieren sowie lösen und ist wirklich vielfältig verwendbar. Man kann mit diesem Fuchs auch normal reden so wie Probleme im echten Leben besprechen und er hilft einem. Wirklich sehr gut diese App kann ich nur weiter empfehlen, gerade für Menschen die etwas länger brauchen etwas zu verstehen!
Lena M
Android user
Ich finde Knowunity ist eine super App. Für die Schule ist sie ideal , wegen den Lernzetteln, Quizen und dem AI. Das gute an AI ist , dass er nicht direkt nur die Lösung ausspuckt sondern einen Weg zeigt wie man darauf kommt. Manchmal gibt er einem auch nur einen Tipp damit man selbst darauf kommt . Mir hilft Knowunity persönlich sehr viel und ich kann sie nur weiterempfehlen ☺️
Timo S
iOS user
Die App ist einfach super! Ich muss nur in die Suchleiste mein Thema eintragen und ich checke es sehr schnell. Ich muss nicht mehr 10 YouTube Videos gucken, um etwas zu verstehen und somit spare ich mir meine Zeit. Einfach zu empfehlen!!
Sudenaz Ocak
Android user
Diese App hat mich echt verbessert! In der Schule war ich richtig schlecht in Mathe und dank der App kann ich besser Mathe! Ich bin so dankbar, dass ihr die App gemacht habt.
Greenlight Bonnie
Android user
Ich benutze Knowunity schon sehr lange und meine Noten haben sich verbessert die App hilft mir bei Mathe,Englisch u.s.w. Ich bekomme Hilfe wenn ich sie brauche und bekomme sogar Glückwünsche für meine Arbeit Deswegen von mir 5 Sterne🫶🏼
Julia S
Android user
Also die App hat mir echt in super vielen Fächern geholfen! Ich hatte in der Mathe Arbeit davor eine 3+ und habe nur durch den School GPT und die Lernzettek auf der App eine 1-3 in Mathe geschafft…Ich bin Mega glücklich darüber also ja wircklich eine super App zum lernen und es spart sehr viel Heit dass man mehr Freizeit hat!
Marcus B
iOS user
Mit dieser App hab ich bessere Noten bekommen. Bessere Lernzettel gekriegt. Ich habe die App benutzt, als ich die Fächer nicht ganz verstanden habe,diese App ist ein würcklich GameChanger für die Schule, Hausaufgaben
Sarah L
Android user
Hatte noch nie so viel Spaß beim Lernen und der School Bot macht super Aufschriebe die man Herunterladen kann total Übersichtlich und Lehreich. Bin begeistert.
Hans T
iOS user